Definition and transformation principle of WVD and STFT
WVD is a time-frequency distribution widely used in non-stationary signal analysis, which is defined as 
WVD can be regarded as the Fourier transform of the signal's time autocorrelation function. Since it is the second time-frequency of the signal, there must be a cross-interference term for the multi-component signal. At present, many existing time-frequency analysis methods basically compromise between multi-component cross-interference suppression and maintaining signal time-frequency aggregation. In fact, even for single-component signals, WVD has its own interference terms. Survey signal
STFT is a linear representation of the signal, and there is no cross-term interference. It is suitable for multi-component signal analysis, and its resolution performance depends greatly on the choice of window function type and window width. In practice, the signal under investigation is generally non-stationary, but STFT assumes that the signal is approximately stationary within the width of the window function, and its window function type usually selects a low-pass window function, such as Gaussian window, Hanning window, etc. Once the window function is selected, its time-frequency aggregation is determined accordingly. According to the principle of uncertainty, the window function time width and bandwidth cannot be arbitrarily small at the same time, so its time-frequency aggregation is not good, as shown in Figure 2.
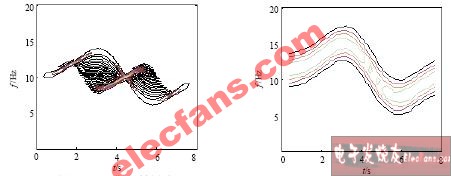
Figure 1 Time-frequency distribution of WVD signal Figure 2 Time-frequency distribution of STFT signal
It can be seen from Figures 1 and 2 that for non-stationary signals, STFT has no self-interference items, but the aggregation is poor, and WVD has good aggregation, but the existing self-interference terms have made the signal itself indistinguishable.Improved analysis method of non-stationary signals
Nantong Boxin Electronic Technology Co., Ltd. , https://www.bosencontrols.com